Λογαριθμικό τριγωνομετρικό ολοκλήρωμα

Tolaso J Kos · #1

Να δειχθεί ότι:

$\displaystyle{\int_0^\infty \frac{\ln \left(1+x^2 \right) \mathrm{arccot}x}{x} \; \mathrm{d}x = \frac{\pi^3}{12}}$

Tolaso J Kos · #2

Επαναφορά!

Tolaso J Kos · #3

Tolaso J Kos έγραψε: ↑
Τετ Ιουν 05, 2019 8:11 pm
Να δειχθεί ότι:

$\displaystyle{\int_0^\infty \frac{\ln \left(1+x^2 \right) \mathrm{arccot}x}{x} \; \mathrm{d}x = \frac{\pi^3}{12}}$

Ξεκινάμε με

λήμματα:

Λήμμα 1: Ισχύει ότι: $\displaystyle{ \int_{0}^{1} \frac{\ln \left ( 1+x^2 \right )}{x} \, \mathrm{d}x =\frac{\pi^2}{24}}$

Απόδειξη: Έχουμε διαδοχικά:

$\displaystyle{\begin{aligned} \int_{0}^{1} \frac{\ln \left ( 1+x^2 \right )}{x} \, \mathrm{d}x &= \int_{0}^{1} \frac{1}{x} \sum_{n=1}^{\infty} \frac{(-1)^{n-1} x^{2n}}{n} \, \mathrm{d}x \\ &=\sum_{n=1}^{\infty} \frac{(-1)^{n-1}}{n} \int_{0}^{1} x^{2n-1} \, \mathrm{d}x \\ &= \frac{1}{2}\sum_{n=1}^{\infty} \frac{(-1)^{n-1}}{n^2} \\ &= \frac{\eta(2)}{2}\\ &= \frac{\left ( 1-2^{1-2} \right ) \zeta(2)}{2} \\ &=\frac{\left ( 1-\frac{1}{2} \right ) \zeta(2)}{2} \\ &=\frac{\zeta(2)}{4} \\ &= \frac{\pi^2}{24} \end{aligned}}$
όπου $\eta$ η συνάρτηση ήτα του Dirichlet και $\zeta$ η συνάρτηση ζήτα του Riemann.

Λήμμα 2: Ισχύει ότι: $\displaystyle{ \int_{0}^{1} \frac{\ln x \arctan x}{x} \, \mathrm{d}x = - \frac{\pi^3}{32}}$ .

Απόδειξη: Έχουμε διαδοχικά:

$\displaystyle{\begin{aligned} \int_{0}^{1} \frac{\ln x \arctan x}{x} \, \mathrm{d}x &= \int_{0}^{1} \frac{\ln x}{x} \sum_{n=0}^{\infty} \frac{(-1)^{n} x^{2n+1}}{2n+1} \, \mathrm{d}x \\ &=\int_{0}^{1} \ln x \sum_{n=0}^{\infty} \frac{(-1)^n x^{2n}}{2n+1} \, \mathrm{d}x \\ &=\sum_{n=0}^{\infty} \frac{(-1)^n}{2n+1} \int_{0}^{1}x^{2n} \ln x \, \mathrm{d}x \\ &=-\sum_{n=0}^{\infty}\frac{(-1)^n}{\left ( 2n+1 \right )^3} \\ &= -\beta(3) \\ &=-\frac{\pi^3}{32} \end{aligned}}$
όπου $\beta$ η συνάρτηση βήτα του Dirichlet.

Λήμμα 3: Για κάθε x>0

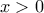Ισχύει $\displaystyle{\mathrm{arccot} \; x + \arctan x = \arctan \frac{1}{x} + \arctan x = \frac{\pi}{2}}$ .

Απόδειξη: Εύκολη και αφήνεται στον αναγνώστη.

Συνδυάζοντας τα παραπάνω τρία λήμματα έχουμε διαδοχικά:

$\displaystyle{\begin{aligned} \int_{0}^{\infty} \frac{\ln \left ( 1+x^2 \right ) \mathrm{arccot}x }{x} \, \mathrm{d}x &= \int_{0}^{1} \frac{\ln \left ( 1+x^2 \right ) \mathrm{arccot}x }{x} \, \mathrm{d}x + \int_{1}^{\infty} \frac{\ln \left ( 1+x^2 \right ) \mathrm{arccot} x}{x} \, \mathrm{d}x \\ &=\int_{0}^{1} \frac{\ln \left ( 1+x^2 \right ) \mathrm{arccot}x }{x} \, \mathrm{d}x + \int_{0}^{1} \frac{\ln \left ( 1+\frac{1}{x^2} \right ) \arctan x}{x} \, \mathrm{d}x \\ &=\int_{0}^{1} \frac{\ln \left ( 1+x^2 \right ) \mathrm{arccot}x }{x} \, \mathrm{d}x + \int_{0}^{1} \frac{\ln \left ( 1+x^2 \right ) \arctan x}{x}\, \mathrm{d}x -\int_0^1 \frac{2 \ln x \arctan x}{x} \, \mathrm{d}x \\ &= \int_{0}^{1} \frac{\ln \left ( 1+x^2 \right ) \left ( \mathrm{arccot} x + \arctan x \right )}{x} \, \mathrm{d}x - 2 \int_{0}^{1} \frac{\ln x \arctan x}{x} \, \mathrm{d}x \\ &= \int_{0}^{1} \frac{\ln \left ( 1+x^2 \right ) \left ( \arctan \frac{1}{x} + \arctan x \right )}{x} \, \mathrm{d}x - 2 \int_{0}^{1} \frac{\ln x \arctan x}{x} \, \mathrm{d}x \\ &=\frac{\pi}{2} \int_{0}^{1} \frac{\ln \left ( 1+x^2 \right )}{x} \, \mathrm{d}x + \frac{\pi^3}{16} \\ &= \frac{\pi^2}{24} \cdot \frac{\pi}{2} + \frac{\pi^3}{16} \\ &= \frac{\pi^3}{12} \end{aligned}}$

Λογαριθμικό τριγωνομετρικό ολοκλήρωμα

Λογαριθμικό τριγωνομετρικό ολοκλήρωμα

Re: Λογαριθμικό τριγωνομετρικό ολοκλήρωμα

Re: Λογαριθμικό τριγωνομετρικό ολοκλήρωμα

Μέλη σε σύνδεση